The platinum resistance thermometer

This type of thermometer, devised by Callendar in 1877, uses the change in the resistance of a platinum wire with temperature to measure the change in temperature. The equation for such a change is:

where <θ is the temperature change and α and β are constants, β being much smaller than α. We therefore ignore the term β² and assume that the resistance of the wire varies uniformly with temperature: a is the temperature coefficient of resistance of the material.
For platinum a = 3.8x10^{-4 o}C^-1.

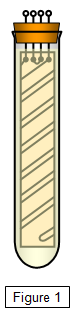
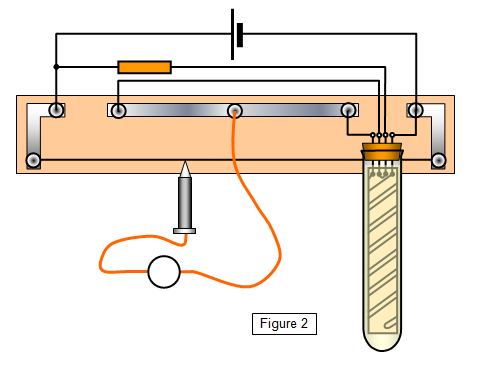
A simple form of the platinum resistance thermometer is shown in Figure 1. It consists of a platinum wire wound non- inductively on a mica former and held in a glass tube by silica spacers. The resistance of the wire is measured with a Wheatstone bridge network and to allow for the change in resistance of the leads a set of dummy leads are included in the opposite arm of the bridge (see Figure 2).

This type of thermometer has a large range, from -200 ^oC to +1100 ^oC and this can be extended by the use of different wires. Bronze has a range starting at -260 ^oC and using carbon temperatures as low as –270 ^oC can be measured.

The advantages of the resistance thermometer are its convenient size, wide range and high sensitivity (±0.000 05 ^oC). It can only be used for steady readings, however, and is not direct-reading.

The accuracy obviously depends on how accurately the bridge can be balanced.